Let x and y be the first and second number, respectively. Then, the statement "adding 4 times the first number and 7 times the second number gives a total of -9" can be written as

and the statement "adding 3 times the first number and 9 times the second number gives -3" can be written as
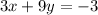
Therefore, the system of equations is:
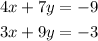
Solving by elimination method.
By multiplying by -3 the first equation and by 4 the second one, we obtain an equivalent system of equations:
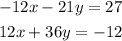
Then, by adding both equations, we get
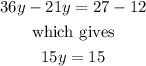
So, y is given by
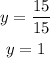
Finally, we can find x by substituting this value into the second equation of our system. It yields,
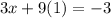
which gives
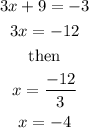
Therefore, the two numbers are: 1 and -4.