Question

The width of a rectangle is 11 units less than the length. If the area is 26 square units then find the dimensions

Answer 1

Length = 13 units

width = 2 units

Explanations:

The formula for calculating the area of a rectangle is given as:

`A=lw`

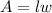
where:

l is the length of the rectangle

w is the width of the rectangle

If the width of a rectangle is 11 units less than the length, then;

`w=l-11`

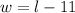
Substitute the expression for the width and the area into the formula for calculating the area.

`\begin{gathered} 26=l(l-11) \\ 26=l^2-11l \\ l^2-11l-26=0 \end{gathered}`

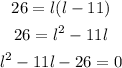
Factorize the resulting quadratic expression

`\begin{gathered} l^2-13l+2l-26=0 \\ l(l-13)+2(l-13)=0 \\ (l+2)(l-13)=0 \end{gathered}`

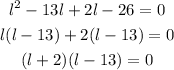
Find the length

`\begin{gathered} l-13=0 \\ l=13units \end{gathered}`

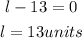
Recall that A = lw, hence;

`\begin{gathered} w=(A)/(l) \\ w=(26)/(13) \\ w=2units \end{gathered}`

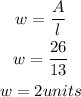
Hence the dimensions of the rectangle is 13 units by 2 units