Question

Cylinder X and Cylinder Y are similar. The volume of Cylinder Y is 89 cm3. Find the volume of Cylinder X.Possible answers:356 cm3712 cm345 cm3178 cm3

Answer 1

We have the following:

We have that the volume of a cylinder is given by the following formula

`V=\pi\cdot r^2\cdot h`

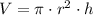
We can calculate the height of the cylinder and, since we know the volume and the radius, so

`\begin{gathered} 89=\pi\cdot2^2\cdot h \\ h=(89)/(3.14\cdot2^2) \\ h=7.09 \end{gathered}`

Since the radius of cylinder x is double that of cylinder y, it means that the height would be double in the same way

`h=7.08\cdot2=14.18`

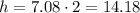
Therefore the volume of cylinder x would be

`\begin{gathered} V=3.14\cdot4^2\cdot14.18 \\ V=712.4\cong712 \end{gathered}`

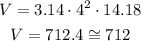
Therefore, the answer is 712 cm^3