1) What we have here is a case for the Similarity of Triangles. Therefore we can find the missing sides by setting a proportion. Like that

1.2) Now let's proceed and find x, based on Similarity we can set another proportion. Considering that y=9.
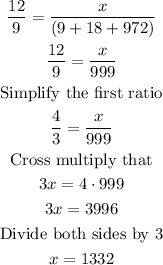
2) So x= 1332 and y =9