Answer: The rate of the boat in still water is 74 km/hr ,and the rate of the current is 19 km/hr
Step-by-step explanation:
Let:
x= the speed of the boat
y=the speed of the stream
Then,
x-y = the speed upstream
x+y = the speed downstream
So, the upstream speed is:
![\begin{gathered} x-y=\frac{165\operatorname{km}}{3\text{hr}} \\ x-y=\text{ 55} \\ \\ \\ \end{gathered}]()
and, the downstream speed is:
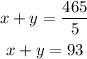
To find the value of x and y, we use the following equations:
x-y= 55
x+y=93
Then, solve for x in x-y =55.
x=55 +y
Substitute x=55 + y into x+y=93.
x+y=93
(55 +y) + y=93
55+2y = 93
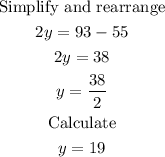
Substitute y=19 into x+y = 93.
x+y= 93
x+ 19 =93
Simplify and rearrange
x=93-19
Calculate
x=74
Therefore, the rate of the boat in still water is 74 km/hr ,and the rate of the current is 19 km/hr.