Activity 1:
We are given two triangles. The two side lengths of one triangle are known but of the other are not. Our task is to choose the value of x and y that will make the triangles congruent.
Now, the side lengths that are congruent are with 31 in the rightmost triangle and 7x -4 in the left-most triangle; therefore, equating them gives
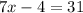
Similarly, side length 24 must equal 4y+8; therefore,
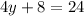
Now we have to choose the values of x and y that will make both equations true.
Let us solve for x in the first equation by first adding 4 to both sides. Doing this gives

Finally, dividing both sides by 7 gives
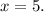
Activity 2:
Now, for the value of y.
To solve for y, we first subtract 8 from both sides to get

Finally, dividing both sides by 4 gives
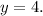
Hence, to conclude x = 5 and y = 4.