Answer:
speed = 7.88 m/s
Step-by-step explanation:
First, we need to find the initial velocity of the stone, so we will use the following equation:
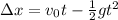
Where Δx is the distance, t is the time, v0 is the initial velocity, and g is the gravity. So, solving for v0, we get:
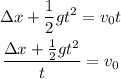
Now, replacing the values, we get;

Then, the velocity of the stone just before it hits the water can be found using the following equation:

Therefore, the speed of the stone is 7.88 m/s