Question

Finish setting up the tangent ratio. X 14 tan(28) = — 289

Answer 1

From the trignometricratio :

The expression for the tangent of an angle of a right angle triangle is express as :

`\tan x=\frac{Opposite\text{ Side}}{Adjacent\text{Side}}`

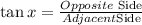
In the given triangle , Angle = 28

The side opposite to the angle 28 is x and the adjacent side to the angle 28 is 14

So, tan 28 is express as :

`\begin{gathered} \tan x=\frac{Opposite\text{ Side}}{Adjacent\text{Side}} \\ \tan x=(x)/(14) \\ x=14\tan 28 \\ x=14*0.5317 \\ x=7.4438 \end{gathered}`

Since tan 28 = 0.5317

Substitute the value of x :

`\begin{gathered} \tan 28=(7.4438)/(14) \\ \tan 28=\text{ 7.44: 14} \end{gathered}`

So, the tan 28 ratio is 7.44 : 14

Answer : tan28 = 7.44/14