To obtain the missing lengths x and y, we would have to use the sine and cosine relations;
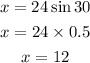
We can move to y;
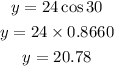
Therefore, the missing lengths are x = 12 and y = 20.78
Part B
We need to find x, x is opposite the 45-degree angle, therefore, we can use the sine relationship;
![\begin{gathered} x=12\sin 45 \\ x=12(\frac{1}{\sqrt[]{2}})=\frac{12}{\sqrt[]{2}}\text{ or 6}\sqrt[]{2} \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/85ga04oplw8zcq2wr9vdsy7rnyi97m6ntd.png)
Therefore, the correct options are A and E