Question

Felipe works in an amusement park and is helping decorate it with strands of lights. This morning, he used a total of 34 strands of lights to decorate 4 bushes and 2 trees. This afternoon, he strung lights on 4 bushes and 4 trees, using a total of 52 strands. Assuming that all bushes are decorated one way and all trees are decorated another, how many strands did Felipe use on each?

Answer 1

Let x and y be the number of strands used to decorate a bush and a tree, respectively. Therefore, the system of equations is

`\begin{cases}4x+2y=34 \\ 4x+4y=52\end{cases}`

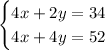
To solve the system using the elimination method, subtract the first equation from the second one; then,

`\begin{gathered} (4x+4y)-(4x+2y)=52-34 \\ \Rightarrow2y=18 \\ \Rightarrow y=9 \end{gathered}`

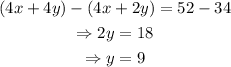
Substitute the last result into the second equation,

`\begin{gathered} y=9 \\ \Rightarrow4x+4y=4x+4\cdot9=4x+36 \\ \Rightarrow4x+36=52 \\ \Rightarrow4x=16 \\ \Rightarrow x=4 \end{gathered}`

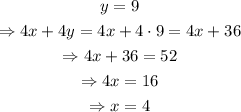
Thus, to decorate every bush, we need 4 strands of lights, and for every tree 9 strands of lights