Question

Find the area of this Parallelogram FGHE. Given: Triangle GJH is an equilateralEJ=20m and GJ=8m

Answer 1

A = 48√3 m²

Step-by-step explanation

Triangle GJH is equilateral, so each side has the same length:

To find the area of the parallelogram we have to find the height, which is the same as the height of the triangle.

We can find H by dividing the triangle into two right triangles as shown above, and applying the Pythagorean theorem:

`8^2=H^2+4^2`

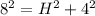
Solving for H:

`\begin{gathered} H=\sqrt[]{8^2-4^2} \\ H=\sqrt[]{64-16} \\ H=\sqrt[]{48} \\ H=4\sqrt[]{3}m \end{gathered}`

The area of a parallelogram is:

`A=B\cdot H=EH\cdot H=12\cdot4\sqrt[]{3}=48\sqrt[]{3}m^2`