Question

If the pumpkin is thrown horizontally what is the launch speed needed to hit the bull’s-eye

Answer 1

Given,

The height of the tower, h=9.0 m

The horizontal distance of the bull's eye from the tower, R=3.5 m

As the pumpkin is thrown horizontally, the vertical component of its initial velocity is zero. And horizontal component is equal to the total velocity of the pumpkin.

From the equation of motion,

`\begin{gathered} h=u_yt+(1)/(2)gt^2 \\ =(1)/(2)gt^2 \end{gathered}`

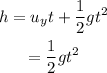
Where u_y is the vertical component of the initial velocity, g is the acceleration due to gravity and t is the time of flight of the pumpkin.

On rearranging the above equation,

`t=\sqrt[]{(2h)/(g)}`

The range of a projectile is given by,

`\begin{gathered} R=u_xt \\ =u\sqrt[]{(2h)/(g)} \end{gathered}`

Where u_x is the horizontal component of the initial velocity and u is the total initial velocity of the pumpkin.

On substituting the known values,

`\begin{gathered} 3.5=u\sqrt[]{(2*9)/(9.8)} \\ \Rightarrow u=\frac{3.5}{\sqrt[]{(2*9)/(9.8)}} \\ =2.58\text{ m/s} \end{gathered}`

Therefore the initial speed needed for the pumpkin to hit the bull's eye is 2.58 m/s