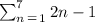We have the following expression 1 + 3 + 5 +... until the 7th term.
Each term equals the previous term plus 2.
So, for any term of number n, we have:
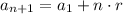
In this case, r = 2.
The sum of all therms of an arithmetic progression with 7 terms and a diference r between two consecutive terms is:

For a1 = 1 and r = 2, we got: