Question

1

Locate the points of discontinuity in the piecewise function shown below.
-(1 + 1)2 + 2; - 1-1 + 2; -1 < a <2
f(1) =
VI - 1;
2 OA X= -1 and 2
OB.
x= 2
OC. x= -1
OD. no points of discontinuity
Reset
Next

Answer 1

Concept

Piecewise functions may be discontinuous at the “breakpoint”, which is the point where one piece stops defining the function, and the other one starts.

The given piecewise function is defined as:

`\begin{gathered} f(x)=-(x+1)^2+2\text{ for -}\infty\text{ }<\text{ x}<\text{ -1} \\ f(x)\text{ = -x + 2 for -1 }\leq x\text{ }<2 \\ f(x)\text{ = }\sqrt[]{x-1}\text{ for }2\text{ }\leq\text{ x }<\infty \end{gathered}`

Let us identify the breakpoints:

There exist two breakpoints at x = -1 and x =2

Next, we take limits to confirm if there is a point of discontinuity at that point.

`\begin{gathered} \lim _(x\to-1^(-1))-(x+1)^2\text{ + 2 = 2} \\ \lim _{x\to-1^(+1)\text{ }}\text{ -x + 2 = }3 \end{gathered}`

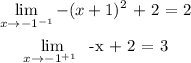
Since the limits are different, there is a jump ate x= -1

`\begin{gathered} \lim _(x\to2^-)-x\text{ + 2 = 0} \\ \lim _(x\to2)\text{ }\sqrt[]{x-1}\text{ =1} \end{gathered}`

Since the limits are different, there is a jump at x =2

Using graphical method

Let us draw a graph of the piece-wise function

We notice that there is a jump at x =-1 and x = 2

Answer: x = -1 and 2 (Option A)