Let's calculate the area and the perimeter of each one.
First, the rectangle 2x8.
The area of a triangle is just the multiplication of the two different sizes of it. In the present case, one is 2 and the other is 8. From this, we are able to calculate as follows:

To calculate the perimeter, we will sum the length of each one of the sides. Because the rectangle has 2 sides with length 2, and the other 2 have length 8, we have the following calculation for the perimeter:
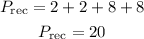
Now, we are able to do the same for the square.
The area of a square is equal to the square of its side. From this, we can calculate it as follows:
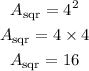
Now, to calculate the perimeter of the square, because each of the sides has the same length, we calculate as follows:
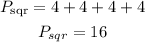
From the above-developed solution, we are able to answer the questions, concluding that both figures have the same area and the rectangle has a larger perimeter.