Question

Last week, Regal Cinemas sold a total of 4,800 movie tickets. Proceedstotaled $32.100. Tickets were sold in 3 ways: a matinee admission cost $5 each; studentadmission cost S6 each, and regular admission cost S8 each How many of each type ofticket was sold if twice as many student tickets were sold as matinee tickets sold? (Solveby setting up a system of the 3 equations in 3 variables)

Answer 1

We will start by drafting out the 3 equations needed in solving this question.

Let matinee ticket be x, student ticket be y, and regular ticket be z.

A matinee admission cost $5 each; student admission cost S6 each, and regular admission cost S8 each and everything summing up to $32100 can be expressed mathematically as:

`5x+6y+8z=\text{ 32100}\ldots\text{.eqn 1}`

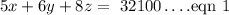
Twice as many student tickets were sold as matinee tickets sold can be expressed mathematically as:

`y=2x\ldots eqn\text{ 2}`

And the total number of tickets can be expressed mathematically as:

`x+y+z=4800\ldots\text{eqn 3}`

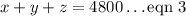
Now we will solve all three equations to know how many of each type of ticket was sold.

Substituting eqn 2 into eqn 1 and eqn 3 we will have:

`\begin{gathered} \text{eqn 1 becomes:} \\ 5x+6(2x)+8z=32100 \\ 5x+12x+8z=32100 \\ 17x+8z=32100\ldots\text{.eqn 4} \\ \\ \text{eqn 2 becomes} \\ x+2x+z=4800 \\ 3x+z=4800\ldots\text{eqn 5} \end{gathered}`

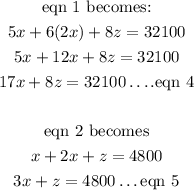
Now we can solve eqn 4 and 5 simultaneously to get the value of x and z

`\begin{gathered} 17x+8z=32100\ldots\text{eqn 4} \\ 3x+z=4800\ldots\text{eqn 5} \\ z=4800-3x \\ \\ \text{eqn 4 becomes:} \\ 17x+8(4800-3x)=32100 \\ 17x+38400-24x=32100 \\ 38400-7x=32100 \\ 38400-32100=7x \\ 6300=7x \\ (6300)/(7)=(7x)/(7x) \\ 900=x \end{gathered}`

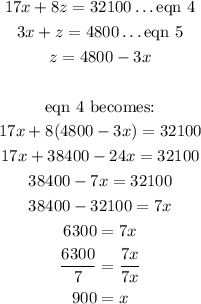
So the number of matinee tickets sold is 900.

`\begin{gathered} z=4800-3x \\ z=4800-3(900)_{} \\ z=4800-2700 \\ z=2100 \\ \\ y=2x \\ y=2(900) \\ y=1800 \end{gathered}`

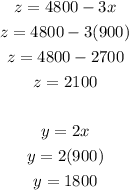
The number of student tickets sold is 1800 and the number of regular tickets sold is 2100.