We assume in this answer a general case (since the question is not complete).
Suppose, we have the next function (a line):
As can we see from the graph, the values for x are all the values in the set of Real numbers. That is, the domain of the function is from -infinity to infinity.
The domain of a function is all the possible values for x in the represented function. That is all the values that this function can take.
On the other hand, the domain of the function:
![f(x)=\sqrt[]{6+x-x^2}](https://img.qammunity.org/2023/formulas/mathematics/college/lp1soha3eoptx190jz55nps79yzas74v5t.png)
It is restricted to values that comply with the next restriction (to be possible in the set of the Real numbers):
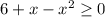
And solving for this, we have that the domain x must be greater or equal to -2 and less or equal to 3 or:
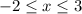
The graphed function is sketched as follows: