Question

all of these questions are together so when you answer question one and go to question 2 question one helps with it and so do the other problems

Answer 1

1. Knowing that Question 1 was answered in the previous session, you know that the number of cubic inches of ice cream that will fit inside the cone is (approximately)

`13.92\text{ }in^3`

2. Given that that the scoop of ice cream that can be modeled by a sphere has this radius:

`r=1.7\text{ }in`

You can find its volume by applying the formula for calculating the volume of a sphere:

`V_s=(4\pi r^3)/(3)`

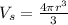
Where "r" is the radius.

Then, by substituting the radius into the formula and evaluating, you get:

`V_s=\frac{4\pi(1.7\text{ }in)^3}{3}`
`V_s\approx20.58\text{ }in^3`

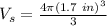
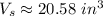
3. Since you need to fill the cone with ice cream and then add the scoop on top, you can find the total volume of ice cream (in cubic inches) in one order by adding the volumes found in Questions 1 and 2 (the number of cubic inches that will fit in a cone and the volume of the sphere of ice cream on the top):

`V_(total)=13.92\text{ }in^3+20.58in^3\approx34.5\text{ }in^3`

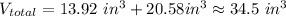
4. You know that tubs, where the ice cream is packed, are cylindrical and have this radius (r)and height (h):

`\begin{gathered} r=4.9\text{ }in \\ h=11.6\text{ }in \end{gathered}`

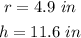
You can find the volume of each tub by using the formula for calculating the volume of a cylinder:

`V_c=\pi r^2h`

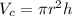
Where "r" is the radius and "h" is the height.

Then, when you substitute values and evaluate, you get:

`V_c=\pi(4.9\text{ }in^2)(11.6\text{ }in)\approx874.98\text{ }in^3`

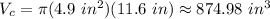
In order to calculate the number of orders that can be made using one tub, you need to divide the volume of one tub by the total volume of ice cream needed to make one order:

`\frac{874.98\text{ }in^3}{34.5\text{ }in^3}\approx25`

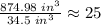
Hence, the answers are:

1.

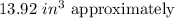
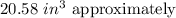
`13.92\text{ }in^3\text{ \lparen approximately\rparen}`

2.

`20.58\text{ }in^3\text{ \lparen approximately\rparen}`

3.

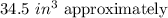
`34.5\text{ }in^3\text{ \lparen approximately\rparen}`

4.

`25\text{ }orders`