Question

explaining volume Formulas: TutorialPart BWhat relationship between the circumference and the diameter is suggested by your observations?

Answer 1

The circunference of a circle is given by:

`C=2\pi r=\pi d`

where r is the radius of the circle and d is the diameter (d = 2r).

So, we can see that the circunference of a circle is equal to its diameter times a constant number (even though pi has an infinte number of decimals, it is a constant number)

* Note:

I'm guessing here, but given the question, I think that what you may do is drawing some circles with different diameters, then mesure their circumferences, an see that the following holds:

`(C_1)/(d_1)\text{ = }\frac{C2_{}}{d_2}\text{ =}(C_3)/(d_3)\text{ = }\ldots.\text{ for as many circles as you draw}`

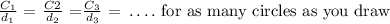
Where C_1 is the first circle circumference and d_1 is its diameter, and so on and so furth.