Answer
6π
Explanation
The area of the circular ripple is calculated as follows:
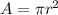
where r is the radius of the circle.
To find how fast is the area of the circular ripple increasing, we need to compute the derivative of the area with respect to time as follows:
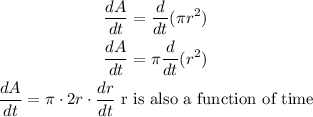
Given that the radius of the circle increases at the rate of 1 foot per second, then:

Considering the rate of 1 foot per second, then after 3 seconds the radius will be:
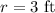
Substituting these values into the formula of dA/dt, we get:
