Question

Han and Clare are stuffing enveloppes. He can stuff 20 enveloppes in one minutes and Clare can stuff 10 enveloppes in one minute. They start working together on a pile of 1000 enveloppes. How long does it take them to finish the pile.

Answer 1

Step-by-step explanation:

According to the problem, Han and Clare are stuffing envelopes. He can stuff 20 envelopes in one minute and Clare can stuff 10 envelopes in one minute. This means that they can jointly stuff 30 envelopes in one minute.

Now, mathematically we know that the number of time it will take to finish stuffing would be:

`\frac{number\text{ of envelopes}}{joint\text{ rate}}\text{ =}\frac{1000\text{ envelopes}}{30\text{ envelopes per minute}}`

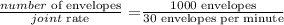
If we simplify this expression, we get:

`\frac{100\text{ envelopes}}{3\text{ envelopes per minute}}`

this can be written as:

`33\text{ + }(1)/(3)\text{ minutes}`

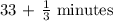
this is true since:

`33\text{ + }(1)/(3)=\frac{33(3)\text{ + 1}}{3}=\frac{99\text{ + 1}}{3}=(100)/(3)\text{ minutes}`

thus, we obtain that it will take them 33 minutes and 20 seconds

Then, we can conclude that the correct answer is:

Answer:

33 minutes and 20 seconds