The given function is,
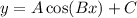
The curve passes through the point (0,8), (60,78) and (120,8).
Substituting the point (0,8) in the given equation,
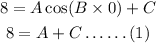
Substituting the value (60, 78) in the given equation,

Substituting the value (120,8) in the given equation,
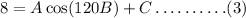
Substracting equation (3) from equation (1),
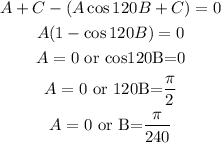
The value of A cannot be zero as the curvature won't be of the cosine.
Thus, the value of B will be pi/240.
Substituting the value of B in the equation (2),
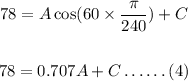
Substracting equation (4) from (1),
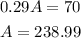
Substituting the value of A in the equation (1),
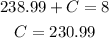
Thus, the required value of A is 238.99, the value of B is pi/240 and the value of C is 230.99.