Question

We started on a new module, but I'm lost on it.

Answer 1

`\begin{gathered} A)20m \\ 25m^2 \\ \end{gathered}`

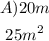
Step-by-step explanation

the distance between 2 points, A and B, is given by:

`\begin{gathered} d=\sqrt[]{(x_2-x_1)^2+(y_2-y_1)^2} \\ \text{where} \\ A(x_1,y_1)andB(x_2,y_2) \end{gathered}`

Step 1

name the other coordinates for the square

A(7,9)

B(10,5)

C(3,6)

D(6,2)

Step 2

find the distances

1)AB

`\begin{gathered} d=\sqrt[]{(10-7)^2+(5-9)^2} \\ d=\sqrt[]{(3)^2+(-4)^2} \\ d=\sqrt[]{9+16\text{ d= }\sqrt[]{25}} \\ \text{distace AB=5} \end{gathered}`

AB=5

2) BD

`\begin{gathered} d=\sqrt[]{(6-10)^2+(2-5)^2} \\ d=\sqrt[]{(-4)^2+(-3)^2} \\ d=\sqrt[]{16+9}=\sqrt[]{25} \\ d=5 \end{gathered}`

DB=5

Step 3

the area of a square is given by

`\begin{gathered} \text{Area}=side^2 \\ \text{replace} \\ \text{Area}=(5m)^2 \\ \text{Area}=25m^2 \end{gathered}`

Step 4

the perimeter of a square is given by:

`\begin{gathered} \text{Perimeter}=4\cdot\text{ side} \\ \text{replace} \\ \text{Perimeter}=4\cdot\text{ 5 ms} \\ \text{Perimeter}=20\text{ m} \end{gathered}`