Question

Determine whether the two lines given below are Parallel, perpendicular, or neither.A) ParallelB) PerpendicularC) Neither

Answer 1

We can determine whether two lines are parallel, perpendicular, or neither using the slopes of the lines.

Two lines are parallel when both of the lines have the same slope, for example:

`\begin{gathered} y=-2x+3 \\ y=-2x-4 \end{gathered}`

Two lines are perpendicular to each other when the product between the lines is equal to -1, for example:

`\begin{gathered} y=-2x+5 \\ y=(1)/(2)x+3 \end{gathered}`

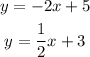
if none of the conditions stated before the lines are neither parallel nor perpendicular.

In the given exercise:

`\begin{gathered} y=-(1)/(3)x+2 \\ y=3x-5 \end{gathered}`

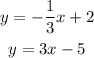
both lines have different slopes, then they are not parallel.

Find the product between the slopes,

`\begin{gathered} m_1\cdot m_2 \\ -(1)/(3)\cdot3 \\ -1 \end{gathered}`

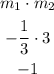
Answer:

The lines shown are perpendicular to each other because the product between the slopes is equal to -1.