Weight of the piece of aluminium is 5.3 lbs
Step-by-step explanation:
We consider the aluminium as a rectangular prism with a cylindrical hole
The formula for volume of a rectangular prism:
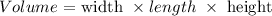
When we look at the aluminium, we see that has inner opening aside the cylindrical hole. The volume of the aluminium without the cylindrical hole:
![\begin{gathered} \text{Volume of the opened prism= volume of outer rectangular prism - Volume of the inner rectangular prism} \\ \end{gathered}]()

![\begin{gathered} \text{Volume of the opened prism without the hole = 270 - 210} \\ \text{Volume of the opened prism without the hole = }60in^3 \end{gathered}]()
The opened aluminuim piece with the cylindrical hole:
Volume of aluminium piece = volume of opened prism - volume of cylindrical hole


Density of the aluminium piece = 0.0974 lbs/cubic inch
Volume of the aluminium piece = 54.4675 in³
weight = ?
