5Let it be L for the weight of the Large box, while S is the weight of the small one. We know that the total weight of the Large boxes is equal to the number of Large boxes times the weight of a single one. The same logic is applied to the small ones. Then, we also know that the total weight of the delivery is equal to the sum of the weight of the Lage boxes with the weight of the small ones.
From this, we rewrite the statements in a math form, as follows:
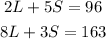
The present problem consists of a system of equations. To solve it, we will multiply the first by 4 and subtract it from the second one, as follows:
![\begin{gathered} 4*1st\to4*(2L+5S=96) \\ 8L+20S=384 \\ 4*1st-2nd\to8L+20S-(8L+3S)=384-163 \\ 17S=221 \\ S=(221)/(17) \\ S=13\operatorname{kg} \end{gathered}]()
From the above-developed solution, we already know that the small boxes have a weight of 13 Kg.
Now, we can substitute it in any of the initial equations to find the weight of the Large boxes. We will substitute in the first on, as follows:
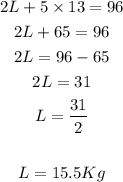
From the solution developed above, we are able to conclude that:
Weight of each large box: 15.5 kilogram(s)
Weight of each small box: 13 kilogram(s)