The basketball tema score 18 points in first 10 minutes, and scored at same rate for 10 minutes means that taem score 18 more points in next 10 minutes.
Determine the points scored by the basketball team in 20 minutes.
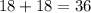
So basketball team score 36 points in 20 minutes.