Question

The dollar value v(t) of a certain car model that is t years old is given by the following exponential function. v(t)=29,900(0.78)^tFind the initial value of the car and the value after 12 years.

Answer 1

(a)Initial Value: $29,900

(b)Value after 12 years: $1516.37

Explanation:

Given the dollar value v(t) of a certain car model that is t years old as:

`v\mleft(t\mright)=29,900\mleft(0.78\mright)^t`

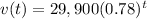
(a)Initial Value

At initial value, that is, when the car was just purchased, t=0

`\begin{gathered} v\mleft(t\mright)=29,900\mleft(0.78\mright)^t \\ \implies v\mleft(0\mright)=29,900\mleft(0.78\mright)^0=29,900*1=29,900 \end{gathered}`

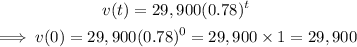
The initial value of the car is $29,900.

(b)The value after 12 years.

When t=12

`\begin{gathered} v\mleft(t\mright)=29,900\mleft(0.78\mright)^t \\ \implies v\mleft(12\mright)=29,900\mleft(0.78\mright)^(12)=\$1516.37 \end{gathered}`

The value of the car after 12 years is $1516.37.