To solve the question given
We will follow the steps below
First, the surface area is given to be 628.32
diameter = 16 this implies that radius = d/2 = 16/2 = 8
First, using the formula
surface area of a cone = πr² + πrl
we will find l
remember that π= 3.14
substituting the values into the above formula
surface area of a cone = πr² + πrl
628.32 = 3.14(8)² + 3.14(8)l
Next is to solve for l
628.32 = 200.96 +25.12 l
subtract 200.96 from both-side of the equation
628.32 - 200.96= 200.96 - 200.96 +25.12 l
427.36 = 25.12 l
Divide both-side of the equation by 25.12
427.36/25.12 = 25.12 l/25.12
17.01 = l
slant height (l) = 17.01
We can now calculate the volume of the cone using the formula:
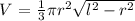
substituting r=8 π = 3.14 and l = 17.01 in the above formula
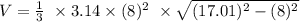
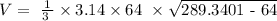
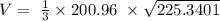
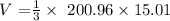
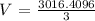
V=1005.47
Volume of the cone is 1 005.47