The formula to be using is as follows:

But A is the total amount, that is, the initial amount plus the interest amount. If we want just theinterest, I, we need to substract the initial amount:
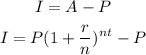
The given information are:
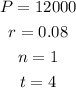
Where r was converted from percentage to decimal and n is 1 because it is compounded only once per year.
So, substituting these values, we have:
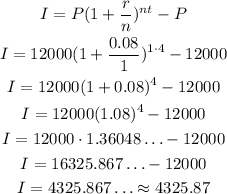
So, the interest amount is approximately $4,325.87.