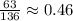Let A be the event that exactly one of the sampled tiles is defective.
In a batch of 17 tiles, 7 are defectives.
3 tiles are sampled at random.
3 tiles can b eselected from 17 tiles in
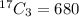
ways.
Therefore, the number of points in sample space is 680.
Now, 1 defective item can be selected from 7 defetctive items in 7 different ways. For each of these ways, remaining two items can be selected from (17-7)=10 non-defective items in
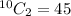
ways.
Therefore, from the batch of 17 tiles, 3 items can be selected so that exactly one item is defective in
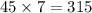
ways.
Therefore, total number of points in sample space in favour of the event A is 315.
By classical definition of probability,
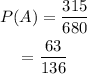
Hence, the probability that exactly 1 of the sampled tiles is defective is