The function composition is defined as shown below
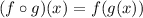
Thus,

And we cannot completely calculate (f∘g)(-2) since we are missing the value of f(-4).
On the other hand, we can calculate the product (fg)(-2) as shown below.
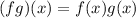
Then,

The result of the product of functions (fg)(-2) is 4.