The 15ft ladder is leant at the top of a wall
The base of the ladder is 5ft from the wall
You have to calculate the heigth of the wall.
First step is to draw the ladder agains the wall:
As you can see the wall, floor and ladder form a right triangle. To calculate the height of the wall you can apply the Pythagoras theorem that states that the square of the hypothenuse is equal to the sum of squares of the base and the heigth of the right triangle:

a= base of the triangle (floor)
b= height of the triangle (wall)
c= hypothenuse (ladder)
From this you can calculate the heigth of the truangle as:
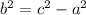
Replace the expression with the given lengths
![\begin{gathered} b^2=(15)^2-(5)^2 \\ b^2=200 \\ b=\sqrt[]{b^2}=\sqrt[]{200} \\ b=14.14ft \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/96pd631s90yjnvntchd3fua4j2yzlk64d1.png)
The wall is 14.14ft tall