The information given can be represented in the diagram below.
This shows that the ellipse cuts across both sides of the x axis at -9 and +9 to give 18 units wide and the y axis at -4 and 4 to give 8 units high.
The equation of the ellipse is
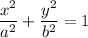
"a" is the distance between the origin and where the ellipse cuts across the x axis.
"b" is the distance between the origin and where the ellipse cuts across the y axis.
Therefore, a=9 and b=4
Hence, the equation of the ellipse gives;
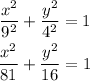
In conclusion, the answer is B