QUESTION A
The company charges a flat fee (constant fee) of $3.00 and $0.75 per mile (variable fee depending on the number of rides).
If x represents the number of miles and y represents the total cost, the equation relating the two variables, and can be used to find the cost of a taxi ride is given to be:
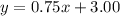
QUESTION B
The cost of a taxi ride for 8 miles can be calculated by putting x = 8 in the equation:

The cost of the taxi will be $9.00.
QUESTION C
If the ride costs $15, we can calculate the number of miles as follows:
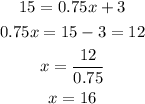
The number of miles traveled is 16 miles.