Law of Sines
Part A
Sine theorem
We are going to use in this problem the Law of Sines:

This is, in any triangle if we divide a side with its opposite angle sine,we will obtain the same result.
Sine theorem applied to this case
In this case:
the side h and the angle tº are opposite
and
the side y and the angle ∠LGM are opposite
then,
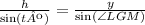
Finding the angle ∠LGM
Using the given information, we have that the angles sº and tº are complementary, this means that their together form a 90º angle:
sº + tº = 90º
then
sº = 90º - tº
Since the sum of all the inner angles of a triangle is 180º, and we have that ΔLGM is a right triangle, then
∠LGM + tº + 90º = 180º
then for ∠LGM, we have that
∠LGM = 180º - 90º - tº
∠LGM = 90º - tº
then ∠LGM and sº are the same angle: 90º - tº
∠LGM = sº
We can replace sº by ∠LGM in the equation we found:
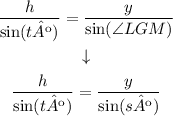
If we multiply both sides by sin(tº), we have:
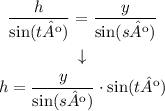
Answer A: h = y · sin(tº)/sin(sº)
Part B
We have that y = 3m, and sº = 38º
in order to find h using the equation we found, we must find tº. Since sº and tº are complementary,
sº + tº = 90º
then
tº = 52º
Now, we can replace in the equation:
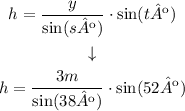
since
sin(52º) ≅ 0.79
and
sin (38º) ≅ 0.62
then
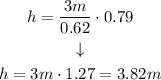
Then, the height of the tree would be 3.82m
Answer B: 3.82 meters