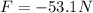We have
We have
Q = magnitude of charge at each of the three locations A, B and C=3.5 x 10⁻⁶ C
r₁ = distance of charge at origin (A) from charge at B = 6cm = 0.06 m
r₂ = distance of charge at origin from charge at C = 7cm=0.07m
F₁ = magnitude of force by charge at B on charge at origin
F₂ = magnitude of force by charge at C on charge at origin
Let's calculate F₁
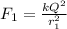
k is a constant that is 9x10^9
we substitute the values
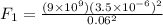
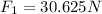
then for the force with charge C
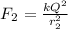

Then we calculate the net force
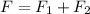
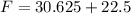
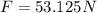
As we can see in the diagram the forces go to the left therefore the solution is