Bank A:
There are no annual fees, so we only need to consider the monthly interest.
The initial unpaid balance is $970; therefore,
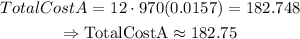
Where 0.0157=1.57% and the 12 in the exponent is there because of the 12 months that a year consists of. The answer is $182.75, bank A.
Bank B:
We need to do something similar to the previous step, the only changes are the interest rate and the annual fee; therefore,
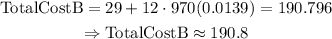
The answer is $190.8, bank B