Given:
Let the cost of one pound of ground sirloin = x
And, the cost of one pound of flank steak = y
A butcher buys 75 pounds of ground sirloin and 50 pounds of flank steak for a total cost of $362.50
So, 75x + 50y = 362.5
A second purchase, at the same price, includes 30 pounds of ground sirloin and 40 pounds of flank steak for a total cost of $200
So, 30x + 40y = 200
So, we have the following system of equations:
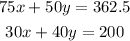
The solution to the system will be as follows:
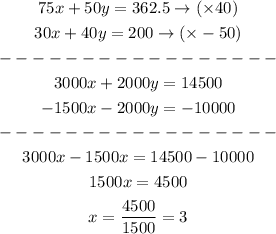
Substitute with x into the first equation to find y
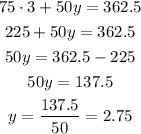
So, the answer will be:
The cost of one pound of ground sirloin = x = $3
The cost of one pound of flank steak = $2.75