Answer:

Step-by-step explanation:
Here, we want to find the time the rocket will hit the ground
What we have to do is to substitute 0 for the height of the rocket y and solve the quadratic equation that results
That simply means we are solving for:
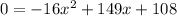
We can use the quadratic formula to solve this
![x\text{ = }\frac{-b\pm\sqrt[]{b^2-4ac}}{2a}](https://img.qammunity.org/2023/formulas/mathematics/college/g6chm4j4qtch7yszwcwrt6mnkvwotsyyab.png)
where a is the coefficient of x^2 which is -16
b is the coefficient of x which is 149
c is the last number which is 108
Substituting the values, we have it that:
![\begin{gathered} x\text{ = }\frac{-149\pm\sqrt[]{149^2-4(-16)(108)}}{2(-16)} \\ \\ x\text{ = }\frac{-149\pm\sqrt[]{29113}}{-32}\text{ } \\ \\ x\text{ = }\frac{-149-170.625\text{ }}{-32} \\ or\text{ } \\ x\text{ = }\frac{-149_{}+170.625}{-32} \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/e10q5eanxyugmlufwhr5ksxmatabhyyxzv.png)
Now, we proceed to get the individual x-values:

Since time cannot be negative, we use the first value only