If he starts running 1 3/4 km the initial day, and then adding 1/2 km each day, we can model this as a linear function.
The first day he rans 1 3/4 km.
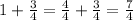
The second day he will run 1/2 km more, so this will be 1 3/4 + 1/2:
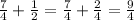
The n-th day he will run:
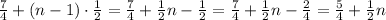
NOTE: n is the index of the day: Day 1 corresponds to n=1, Day 2 correspond to n=2 and so on.
So we have to find at which day he reaches 5 km:
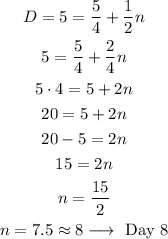
Answer: he will reach the goal at Day 8.