We want to determine the slope of the equation;

We need to express the equation in the slope-intercept form;

where m is the slope and c is the intercept.
Firstly, let's multiply the equation through by 3;
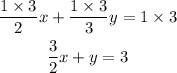
Then, let's subtract (3/2)x from both sides;

comparing the resulting equation to the slope-intercept form of straight line equation, then the slope is the coefficient of x;
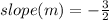
The slope of the line is -3/2