Question

Hi, can you help me answer this question, please, thank you:)

Answer 1

The probability of guessing correctly is 0.55. This means that the probability of not getting it correctly is 1-0.55 or 0.45.

To obtain the probability of guessing all 32 games correctly, substitute 0.55 for p and 0.45 for q in the following formula:

`P=_nC_np^xq^(n-x)`

where P is the missing probability, n is the total number of games played, x is the number of games that were guessed correctly, p is the probability of guessing it correctly, and q is the probability of guessing it incorrectly.

Thus, we obtain the following:

`P=_(32)C_(32)(0.55)^(32)(0.45)^(32-32)`

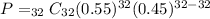
Simplify the expression. Get the value of the combination 32 taken 32, and then simplify the exponent. Evaluate the exponential expression.

`\begin{gathered} P=1(0.55)^(32)(0.45)^0 \\ =(0.55)^(32)(1) \\ \approx0.0000000049159 \end{gathered}`

Therefore, the probability of guessing 32 games correctly is approximately 0.0000000049 or 0.

To obtain the probability of guessing exactly 9 games, substitute 32 for n, 9 for x, 0.55 for p, and 0.45 for q. Simplify the expression. Get the combination and then evaluate the exponential expressions.

`\begin{gathered} P=_nC_np^xq^(n-x) \\ P=_(32)C_9(0.55)^9(0.45)^(32-9) \\ =(28048800)(0.55)^9(0.45)^(23) \\ \approx(28048800)(0.004605366584)(0.0000000105654456) \\ \approx0.001364791497 \end{gathered}`

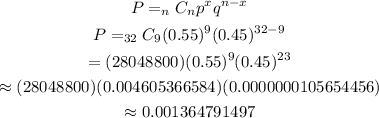
Therefore, the probability of guessing 9 games correctly is approximately 0.0014.

To obtain the probability of guessing exactly 23 games incorrectly, substitute 32 for n, 7 for x, 0.55 for p, and 0.45 for q. Note that we used 7 for x since the value of x is the number of games taht are guessed correctly. Thus, it must be 32-25 which is equal to 7.

Simplify the expression. Get the combination and then evaluate the exponential expressions.

`\begin{gathered} P=_nC_np^xq^(n-x) \\ P=_(32)C_7(0.55)^7(0.45)^(32-7) \\ =(3365856)(0.55)^7(0.45)^(25) \\ \approx(3365856)(0.01522435234)(0.00000000213950273) \\ \approx0.00010963449 \end{gathered}`

Therefore, the probability must be approximately 0.0001.