Question

Over the last 3 evenings, Linda received a total of 130 phone calls at the call center. The third evening, she received 4 times as many calls as the first evening. The first evening, she received 10 fewer calls than the second evening. How many phone calls did she receive each evening? Number of phone calls the first evening: Number of phone calls the second evening: Number of phone calls the third evening:

Answer 1

The Solution:

Given:

`\begin{gathered} Total\text{ calls}=130 \\ Third\text{ evening = 4}* first\text{ evening} \\ First\text{ evening}=\text{ second evening}-10 \end{gathered}`

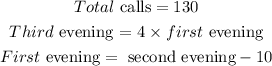
Let:

x = the number of calls received on the first evening.

y = the number of calls received on the second evening.

z = the number of calls received on the third evening.

So,

`\begin{gathered} z=4x \\ x=y-10 \end{gathered}`

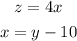
So, we have:

`\begin{gathered} x+y+z=130 \\ \\ x+y+4x=130 \\ 5x+y=130 \end{gathered}`

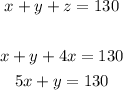
Substitute for x.

`\begin{gathered} 5(y-10)+y=130 \\ 5y-50+y=130 \\ 6y=130+50 \\ 6y=180 \end{gathered}`

Divide both sides by 6.

`y=(180)/(6)=30\text{ calls.}`

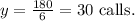
To find x.

`x=y-10=30-10=20\text{ calls}`

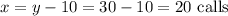
To find z:

`z=4x=4*20=80\text{ calls}`

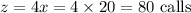
Therefore, the correct answers are:

The first evening = 20 calls

The second evening = 30 calls

The third evening = 80 calls.