Step-by-step explanation
We need to solve the following system of equations:
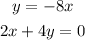
We can use the substitution method. The first equation gives us an expression of y dependent on x. We can take the second equation and replace y by that expression so we obtain an equation with only one variable:
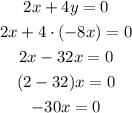
Then we divide both sides by -30:
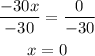
So we have found that x=0. We use this value in the expression of y:
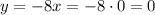
So we have x=0 and y=0.
Answer
Then the answer is (0,0).