Question

Given the points R(6,-2) and T(-9,-7), find the coordinates of point S on RT such that the ratio of RS to ST is 3:2

Answer 1

R(6,-2)

T(-9,-7)

RS:ST = 3:2

To find the x coordinate of the point S we use the next equation:

`X_S=X_R-r(X_R-X_T)`

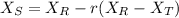
Where the r is the ratio expressed as a fraction

`r=\frac{\text{3}}{5}`

Then:

`X_S=6_{}-(3)/(5)(6_{}-(-9)_{})`
`X_S=6-(3)/(5)(6+9)=6-(3)/(5)(15)=6-(45)/(5)=6-9=-3`

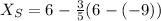
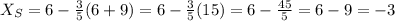
Then the y coordinate of the point S is determined by:

`Y_S=Y_R-r(Y_R-Y_T)`
`Y_S=-2-(3)/(5)(-2-(-7))`
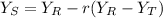
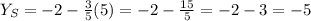
`Y_S=-2-(3)/(5)(5)=-2-(15)/(5)=-2-3=-5`Then so, the coordinated of the point S are:(-3 , - 5)