Answer:
13.391 ft
Explanations:
The schematic diagram of the given question is shown below;
The height of the tree is expressed as:
H = h +5
Determine the value of h using the SOH CAH TOA identity
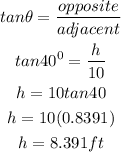
Determine the height of the tree
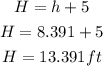
Hence the height of the tree is 13.391ft