Given
Determine if it is an arithmetic or geometric sequence:
72, 48, 24…
Solution
Arithmetic Sequence is described as a list of numbers, in which each new term differs from a preceding term by a constant quantity.
Geometric Sequence is a set of numbers wherein each element after the first is obtained by multiplying the preceding number by a constant factor.
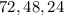
-24 is the constant quantity, therefore it is an arithmetic sequence
Find the 12th term
formula

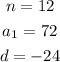
We can plug in to the formula
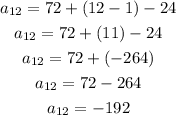
The 12th term of the sequence is -192