Question

Can u help wi tg 7 a and b pls

Answer 1

We can see two functions and we have to find the average rate of change of them from x = 1 to x = 2.

The average rate of change is given by the formula:

`\text{ The average rate of change}=\frac{\text{ change in y}}{\text{ change in x}}=(y_2-y_1)/(x_2-x_1)=(f(x_2)-f(x_1))/(x_2-x_1)`

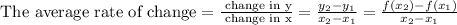
Therefore, to find the average rate of change of a function, we have to find the values of the function for the given values, x1 = 1, x2 = 2, and then find the corresponding ratio as follows:

Finding the average rate of change for the function f(x) = 2x - 5 from x = 1 to x =2

To find it, we can proceed as follows:

1. Find the value of the function for x1 = 1 and x2 = 2:

`\begin{gathered} f(x_2)=f(2)=2(2)-5=4-5=-1 \\ \\ f(x_1)=f(1)=2(1)-5=2-5=-3 \end{gathered}`

2. Then the average rate of change is:

`A_{rateofchange(1\text{ to 2\rparen}}=(f(x_2)-f(x_1))/(x_2-x_1)=(-1-(-3))/(2-1)=(-1+3)/(1)=2`

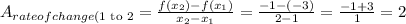
Therefore, the average rate of change for f(x) = 2x - 5 from x = 1 to x = 2 is 2.

Finding the average rate of change for the function f(x) = -3x²+5x-1 from x = 1 to x =2

1. We can proceed in a similar way as before to find the average rate of change in this case:

`\begin{gathered} f(x)=-3x^2+5x-1 \\ \\ f(x_2)=f(2)=-3(2)^2+5(2)-1=-3(4)+10-1=-12+10-1=-2-1 \\ \\ f(x_2)=-3 \end{gathered}`

2. And

`\begin{gathered} f(x_1)=f(1)=-3(1)^2+5(1)-1=-3(1)+5-1=-3+5-1=2-1=1 \\ \\ f(x_1)=1 \end{gathered}`

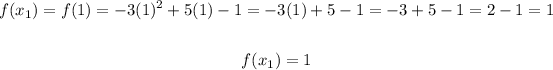
3. Then the average rate of change of this function from x = 1 to x = 2 is:

`\begin{gathered} A_{\text{ rate of change\lparen x =1, x=2\rparen}}=(f(x_2)-f(x_1))/(x_2-x_1)=(-3-1)/(2-1)=(-4)/(1)=-4 \\ \\ A_{\text{ rate of change\lparen x =1, x=2\rparen}}=-4 \end{gathered}`

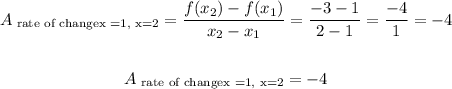
Therefore, in summary, we can conclude that the average rate of change from x = 1 to x = 2 is:

1. For the function f(x) = 2x - 5 is 2

2.For the function f(x) = -3x²+5x-1 is -4