ANSWER and EXPLANATION
We want to transform the system of equations given into an augmented matrix.
First, we have to put the equations in the form:
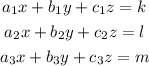
where k, l, and m are constants
a1, a2, a3, b1, b2, b3, c1, c2, and c3 are coefficients of x, y, and z in the equations
Therefore, we have that the equations become:
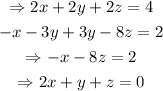
The augmented matrix will be in the form:
From the above equations, we have that the coefficients and constants are:

Therefore, the augmented matrix is: